This is my 3rd year doing this project. Apparently, the 3rd time is the charm. I posted about my experience last year here. You can smell the fear in that post.
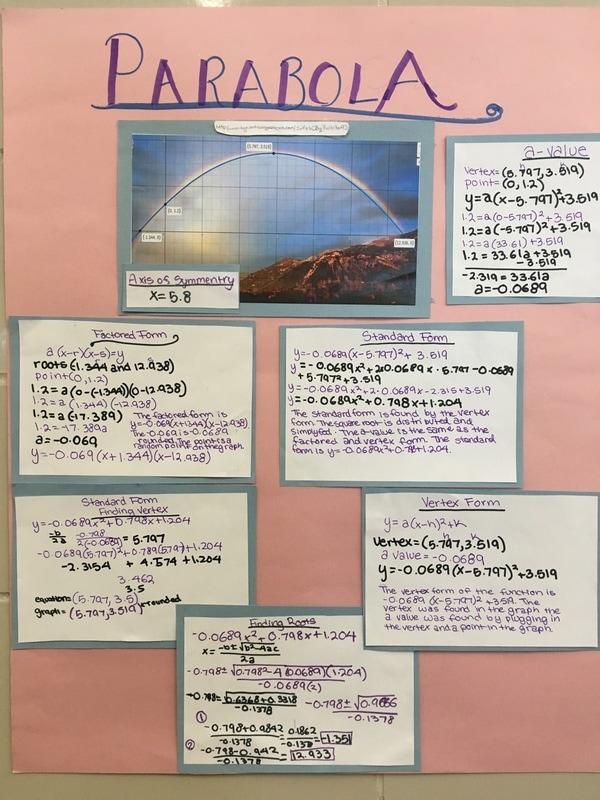
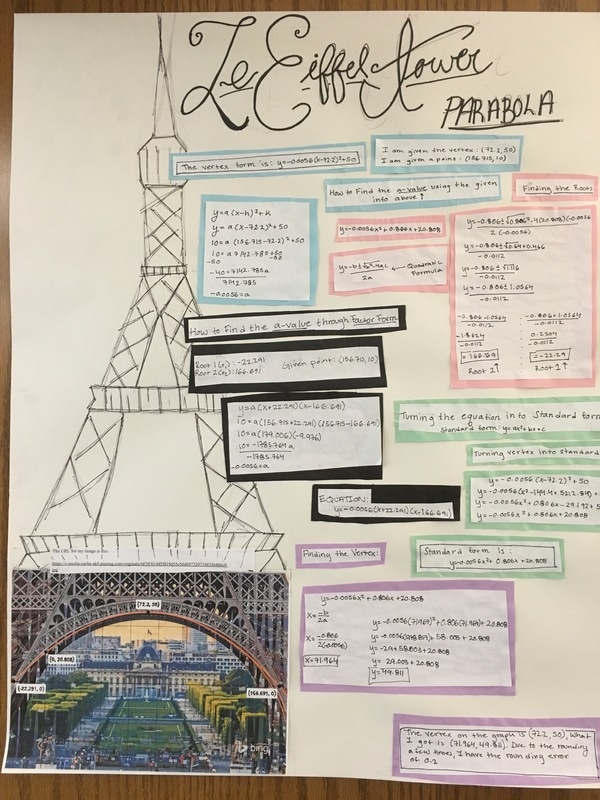
At this point in quadratics, we've covered: graphing, factoring, the quadratic equation, vertex form, and transformations. What I really want is for students to see the connections between multiple representations. I want students to see that you can find the roots from the graph in Desmos. Those roots are the same as the roots in the factored form of the equation. Those roots are also the solutions to the quadratic formula. Slowly, we're making progress.
Here's the rough outline of the project:
1) Find a "real-world" example which you can fit a parabola to. If you want to fit a parabola to Payton Manning's forehead, or Donald Trump's smile, or some other hilarious thing, that's fine with me--you'll just lose the "real-world" point.
2) Fit a parabola to the image using vertex form.
3) Show all points of interest on the Desmos graph (roots, y-intercept, vertex, axis of symmetry)
4) Demonstrate you know how to calculate the a-value for the vertex form of the equation using the vertex and a point.
5) Show me you know how to find the factored form of the equation from your Desmos graph.
6) From either vertex or factored form, calculate standard form of the equation.
7) From the standard form, show me you know how to calculate the vertex of the parabola. It should be the same vertex as your graph.
8) From the standard form, use the quadratic formula to calculate the roots of the equation. These should be the same roots as your graph.
9) Put your name on the back of the poster so I can take pictures of your work and put them online without worrying about student identities (that's one of the reasons at least).
| 10_project_work_before_making_poster.docx |
| Project Directions
| Project Checklist
| ||||
Here are some more student examples to give you an idea of the range of quality to expect. Not everything is super pretty all of the time.


 RSS Feed
RSS Feed



