|
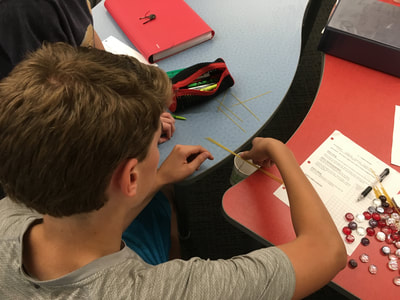
I'm struggling to find time to write thoughtful posts. But I am taking pictures. Here's some fun shots of our Ball Drop experiment in Algebra 1.
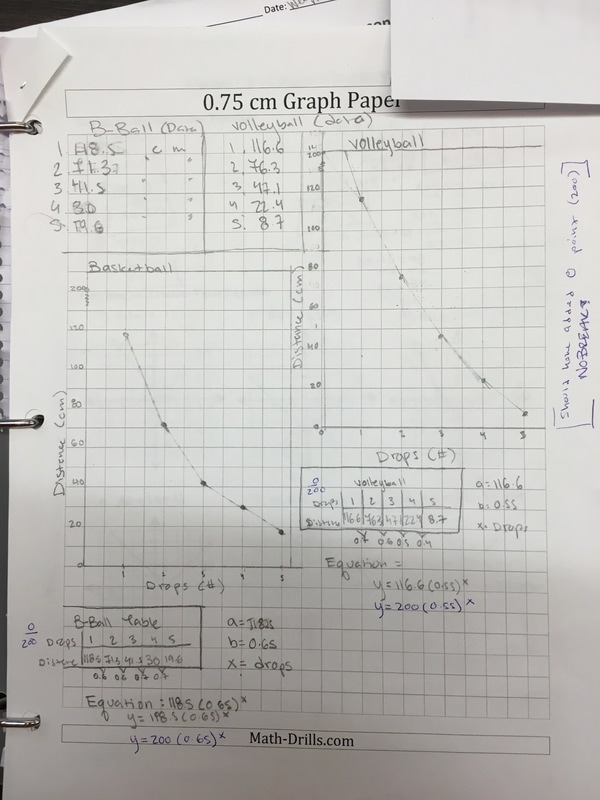
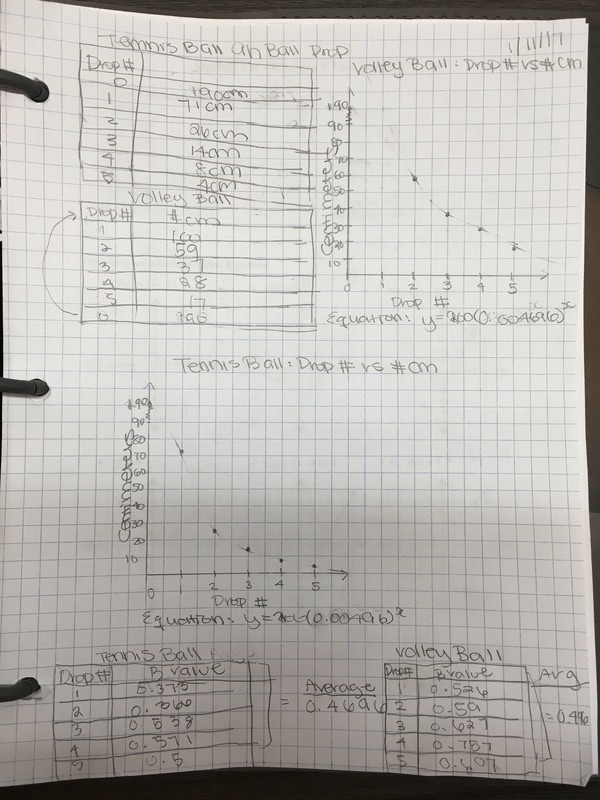
The directions were simple: •Drop a ball from any initial height, measure in cm. •Measure the height for 5 bounces of a ball (I suggest using a table). •Graph the points (graph paper) •Create an equation that models/fits your data. •Do this for two different balls (don’t put both balls on the same graph) The results were fabulous!
Students had great conversations surrounding how to find the constant multiplier if it never ended up being the same between bounces. We also had heated arguments about whether connecting the data points with a curve made sense in the situation. (The students came to the consensus that they did not think the graph would be continuous.) Experiments with pennies and dice exploring exponential growth and decay. 2 days. 500 dice. More pennies than you care to think about. High engagement. Excellent modeling.
From a height just shy of 16 ft (190.5 in), two groups made amazing drops today: Super proud of my classes today.
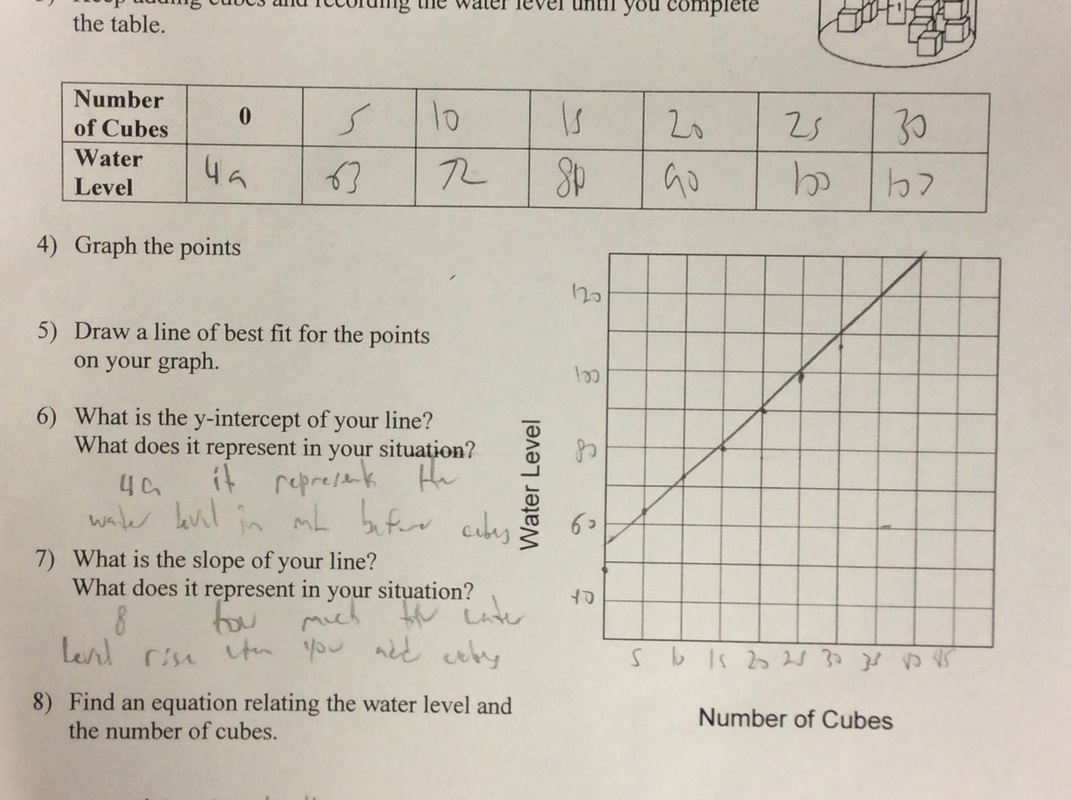
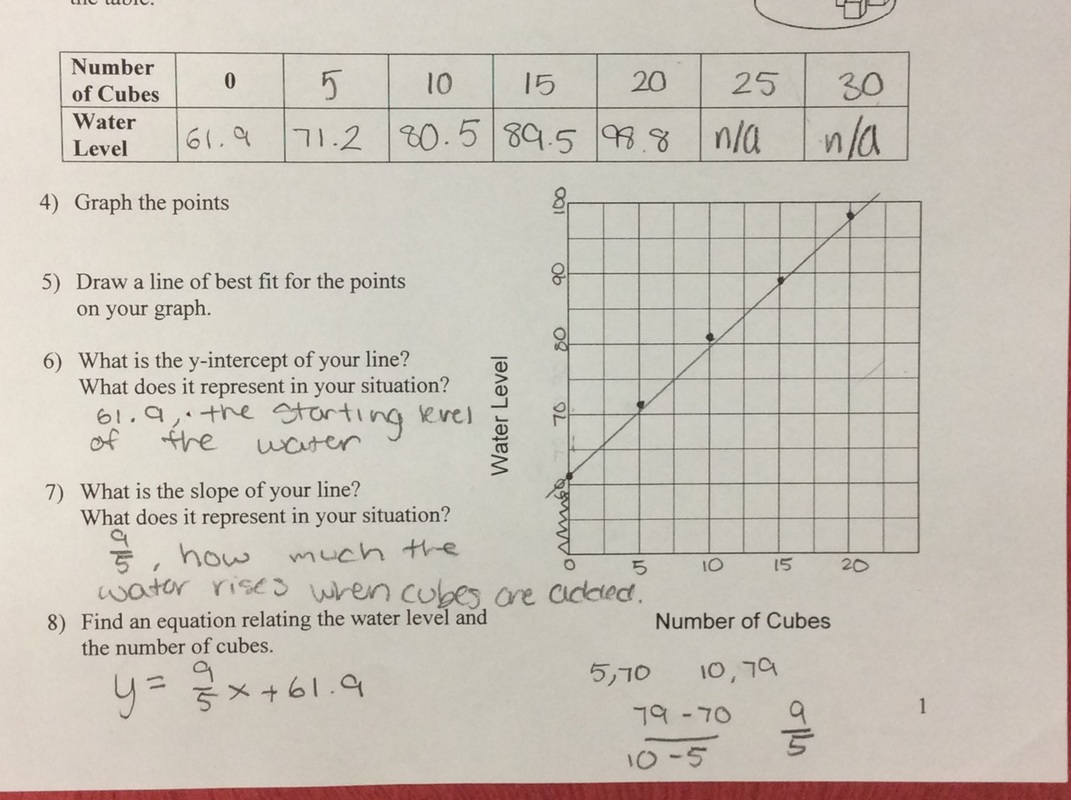
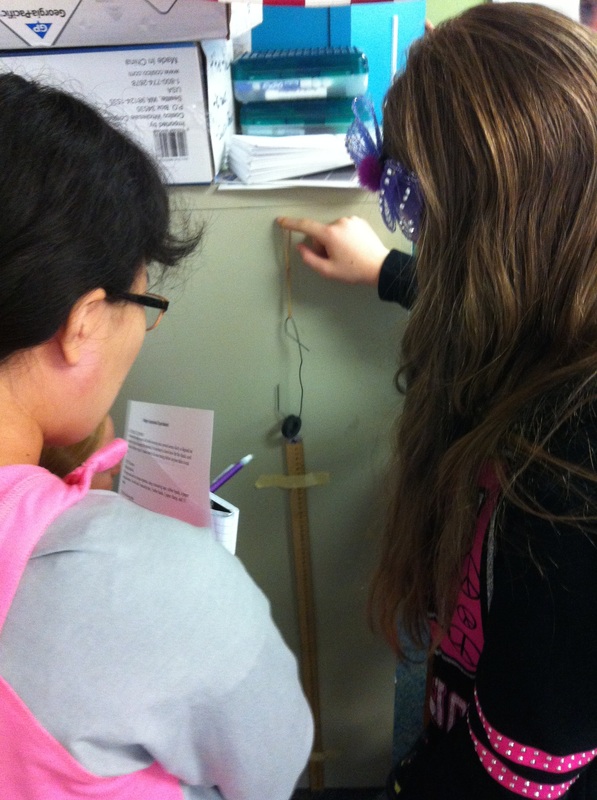
This last week we played with water in Algebra 1. My students have gone through all the typical linear stuff, but they're still weak on creating equations of lines from two points or in describing what certain aspects of the equation mean when representing a situation. To help give some motivation to practice the skill, I have a bunch of experiments in which we gather data, create lines of best-fit, and create equations to model the data. The experiments aren't amazing or anything but they beat a worksheet hands down any day. Here's the gist of how this goes. Students fill up a container with a random amount of water. They stick a ruler into the container and measure the initial height of the water. Students then add an equal amount of either centimeter cubes or glass beads to the container each time and record how the water height changes. Spoiler: the relationship is linear. Since I'm not a science teacher, I don't have all the stuff I need to do experiments just hanging out in my room. I ask students to bring stuff in. I go shopping in the science rooms. And, just in case you're wondering, science teachers actually want to help you make this work. They desperately want students to make the connections between the applications in their subject and the the math students are learning down the hall. Seriously. If I'm honest, it takes more work than I'd like to admit to pull of a good experiment but the increase in student engagement and the rapport it builds with students is amazing. And, it's fun. Fun matters. A lot. I put out a plea for staff and students to bring me glass jars. Within a couple of days I had somewhere around 15-20 jars on my classroom counter. Quick side note: I tried metal containers before but it was too hard to read the water height because of the reflection off the sides of the container. I also opted to use graduated cylinders from the science department this year. However, this put a slight wrinkle in my plans. I planned to use centimeter cubes to displace the water but I want data that isn't perfect. For those of you who aren't up on your unit conversions, 1 cm cubed is equal to 1 mL. That's about as perfect as it gets. To add some variation/spice to the experiment I had students use centimeter cubes with the glass jars and glass beads with the graduated cylinders. Since the glass beads aren't all the same size, it gave at least a little noise to the data. Students did much better when asked to create an equation from their data this experiment. However, when asked about what the slope and y-intercept meant we still had issues. In general, students nailed the y-intercept--it was the initial water height in the container. Students struggled explaining the what the slope meant in the situation. I have to keep reminding myself that what is obvious to me isn't at all obvious to an 8th grader. Here's my handout if you're interested.
This past Friday I had the opportunity to present at my state math conference (#CCTM16). I think I was the only presenter that needed a wagon to bring in all my supplies. Not kidding.
I presented on one of the few things I'm good at--getting students out of their seats and getting students talking to each other. Over the past 4-5 years I've been on a journey to involve more more active learning in my math classes. It's a bit more than just 3-act math or problem-based learning. Don't get me wrong, I love me some Math Assessment Project or Emergent Math. There are all sorts of wonderful things happening in the world of online math teachers that wasn't happening 10 years ago. I totally dig it. But that's not really what floats my boat. I'm interested in students modeling the world around them. All the better if they can also be the ones to run the experiment and collect the data. Basically, as close as my class can get to a physics class--the better. It's probably because I married a physics girl.
So, I presented on what I know; doing experiments in math class. We started out with a quick intro about how this isn't meant as one more thing you can add to your classes. This is meant as a replacement for something you already do in your classes. Math classes in the U.S. already try to do too much. Adding one more thing isn't helpful. Because it would be super awkward to spend 50 minutes talking about active learning without actually doing something we started out with an experiment: ball drop. I had the participants arrange themselves in groups of two or three. After a sudden death round of rock-paper-scissors, the loser came up to get supplies for the group. Here's the slide I put up for directions.
I didn't give much more help than that. Some groups had issues counting the 4th or 5th bounce. For those groups who struggled I made a observation that catching the ball at the top of the bounce and then dropping it would not change the data. I think we were the only session of the day to have participants on the floor or actively using the hallway. Epic!
While groups were busy trying to model the data, I went around the room asking questions and taking pictures of solutions strategies I wanted to discuss as a larger group. When I called the groups back together for a whole group discussion, this happened:
My Reflector2 app stopped working. I have this app that allows my phone to mirror to my computer screen. Normally, it works well. It had worked well the 10 minutes prior to this moment. Curses. Time to do what you would do in a classroom--switch strategies.
We did our best to talk about solution strategies without looking at pictures. The room was very gracious about it. A classroom of students would have revolted.
Then we hobbled through talking about how running a classroom experiment naturally flows into covering the CCSS math practice standards and the Principles to Actions math teaching standards. Again, super hard to make seamless connections without the major component of those connections--student work. But, this was a room full of sympathetic math teachers/coaches and they helped me out.
That's when we finally got to talk about some of the wicked cool experiments we can do in math class without needing a physics lab. Well, at least for most of them. I've got the presentation linked below, so I won't go into details here. The gist of what I wanted to say to them was, it's not too hard. You can do it. Ask the science teacher down the hall for help. For real. Ask her. She will LOVE it that you want to do an experiment and will help you gather supplies. Not joking. The physics teacher will probably even set up a lab for you more than once. Again, not joking.
I've already hear back from one person: "I was inspired enough to try it! I'm going to try to do a lesson with perimeter, area, and volume next week. I stole some boxes, meter sticks, and string from my science teacher next door. You were right, he was excited to help. He already had the boxes made for a lab they used to do. I'm going to try to use different balls for my students who need some more complexity and boxes for the students who need more straight-forward data collection." - Mike That, my friends, is awesome!
All in all, it was a good experience. I didn't die like I thought I was going to. I might even do it again sometime. Except it would have to be on the exact same topic. I feel a bit like a one-trick pony.
Here's the slide deck for the presentation:
AND, you should be super jealous, I ate lunch with this wonderful #MTBoS crew! From the left: @MathEdnet, @lisabej_manitou, @pwharris, @0mod3, and me.
This coming Friday I have the pleasure of sharing some things I do in my class with other math teachers. Super stoked! #CCTM16
I get to talk about something near and dear to my heart--using experiments in math class. Rather than chat people up for 50 minutes, we're going to start off by doing an experiment together. The link is pretty old but you'll get the picture.
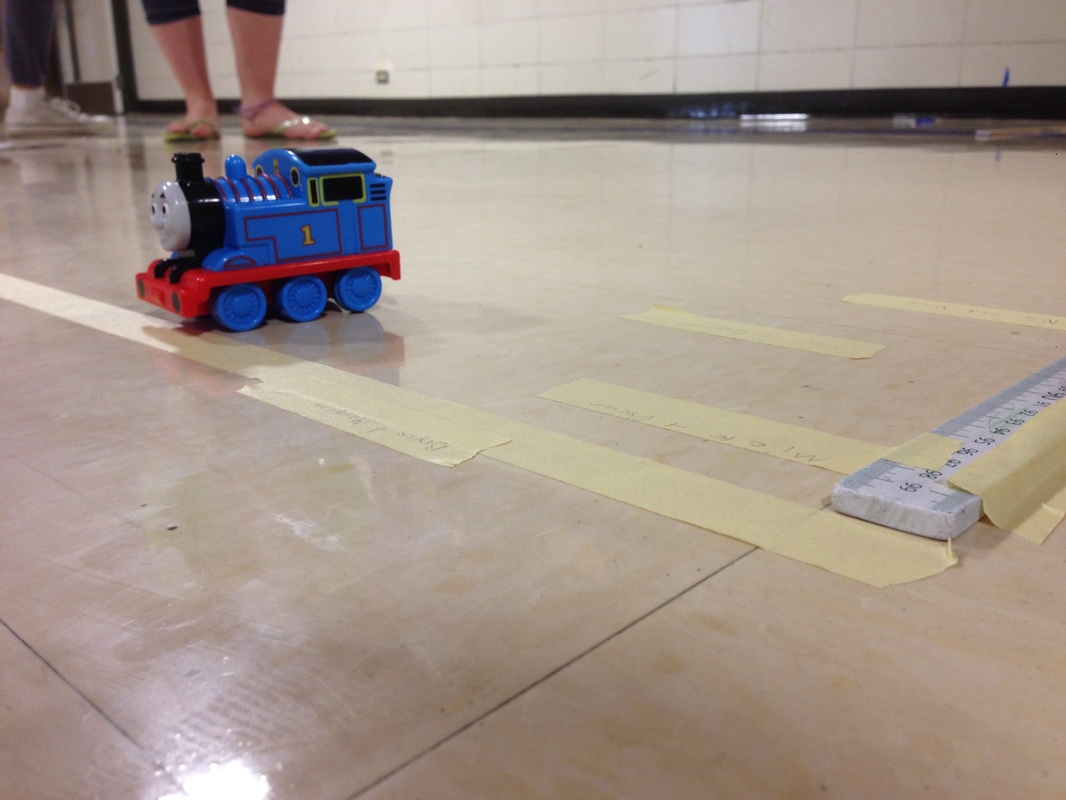
My goal is to give teachers an experience in which they see this type of lesson not simply as an add-on but as a replacement for some of the normal classroom lessons. After modeling exponential decay with bounce height, we're going to do some discussion modeling a la 5 Practices. I really should get a kickback for how often I plug that book. Then we're going to switch into teacher mode and look at the CCSS Math Practice Standards and NCTM's Principles to Actions Math Teaching Practices. It's amazing how naturally you can hit multiple standards--about 5 of the #CCSSmath and 5 of the #NCTMp2a without trying that hard. The rich conversations we have in class after these rich lessons help lay the foundation of conceptual understanding that procedural fluency builds on. If nothing else, I'll have a good time. Here's a link to the presentation. Let's start off with a little reality check. Almost none of what goes on in my classroom is new. I beg, borrow and steal from every brilliant teacher I meet. Barbie bungee has been around at least since the late 1990's--that's when I first encountered it. However, newness or uniqueness has nothing to do with student engagement. Students eat this stuff up! The last time I had a student ask me when we were every going to use this was when we were calculating percentages for tips in a restaurant. Eh, it probably happened when we were looking at rational functions last year but I bet I blocked it out to pretend I was doing a better job with that unit than I actually was. Day 1: For weeks in advance I have students bring in their childhood Barbie dolls. This year I even had some of the staff raid their kids' toy boxes. Even though we do all sorts of other experiments while we wait for the stock of plastic dolls to reach a critical mass, this experiment is what the kids are waiting for. I don't give a lot of directions with this activity. At this point, we've spent the last two weeks looking at messy data. We've gotten really good at making lines of best-fit and then creating an equation from two points on the graph. This is the only slide I put up on the screen. We talk about how I will drop the Barbies come show time--feet touching the bottom of the board. We talk about some way to get Barbie's hair to stay down so we can get good measurements. We talk about being respectful of other classes while we're in the halls. And then they go for it. We spend the rest of the first day gathering data, creating scatter plots, drawing lines of best-fit, creating equations and figuring out how many rubber bands we will need to get as close to the ground as possible. After a couple of years I started requiring some certain work in order to meet my objectives. Each student must: gather data, make a scatter plot, create a line of best fit, find the equation for the line of best fit, and show work for finding the number of rubber bands they want to use. Day 2: On the second day--the day of truth--I begin class by asking for some help. We need: -a videographer (uses my iPad): We use this for instant replay while I'm on the roof. Any disputes get settled quickly by going back to the video. -a photographer (uses my phone): I attended a technology conference years ago. The keynote speaker--whose name escapes me at the moment--said if we don't tell our stories someone else will. It's why I started blogging. It's the day I got a Twitter account. I want my parents and my admin to know the awesome things that go on in my class. I want to steer the conversation in the direction I want it to go rather than hope my principal comes in on a 'good'; day. -2 measurement experts: These are the folks that are in charge of the scoreboard. They corral everyone into putting their group names on a piece of masking tape and then placing the masking tape at the appropriate height on the scoreboard. All while I'm on the roof. And of course, if you give students your devices, they will take selfies. *sigh* I deleted lots of them this year. You don't have to use a roof. I've done it from the ceiling of my class. I use a roof for the spectacle. I want students to feel like this is special compared to what we normally experience in a school day. Plus, the kids from the other classes stare out the windows and wish they were in math class. Love it. My videographers hard at work. Plus a student who had slow motion capabilities on her phone so she volunteered to help out. Here's an example of instant replay on the iPad. I ask students to take screenshots of the close ones so I can fire off an email at the end of the day to parents without having to sift through all of the pictures. One of the side benefits of taking video is that people outside my classroom get to hear the students' reactions to math class. Never underestimate the power of a parent hearing their child squeal with delight in your classroom. I've seen tears of joy on more than one occasion. It's not that I'm a better math teacher than most. I decided to start telling my own story instead of letting my students, my admin, or the broader culture tell it for me. The rest of the world doesn't know the awesomeness that is math class. Racing Day in Algebra 1 Goal: come as close as you can to the finish line without going over. How can you possibly come any closer to the finish line than this group? Wait for it. Wait for it. Oh. My. Goodness. This group of brilliant students got as close to the line as I have ever seen! Congratulations Thomas. That's a photo finish if I ever saw one.
This year I find myself teaching a class called Algebra 1B. It's basically the second half of Algebra 1--except that I have almost the whole year to teach it. So pretty much, almost every activity I've ever collected I get to do with these kiddos. Here's our last two weeks together. Next week: Barbie Bungee Jump
|
Andrew Busch
I teach Math at Ralston Valley High School in Arvada, CO. Categories
All
Archives
March 2019
|
|||||||||||||||||||||||||||
















































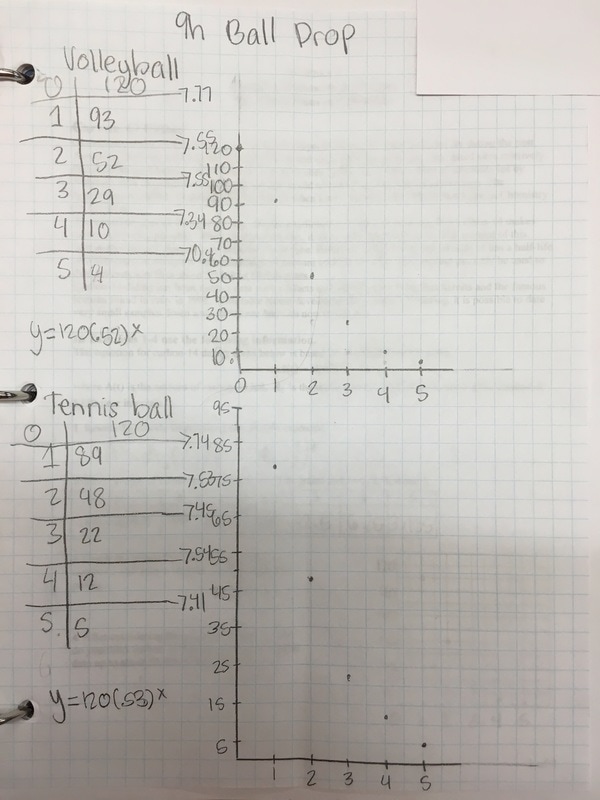
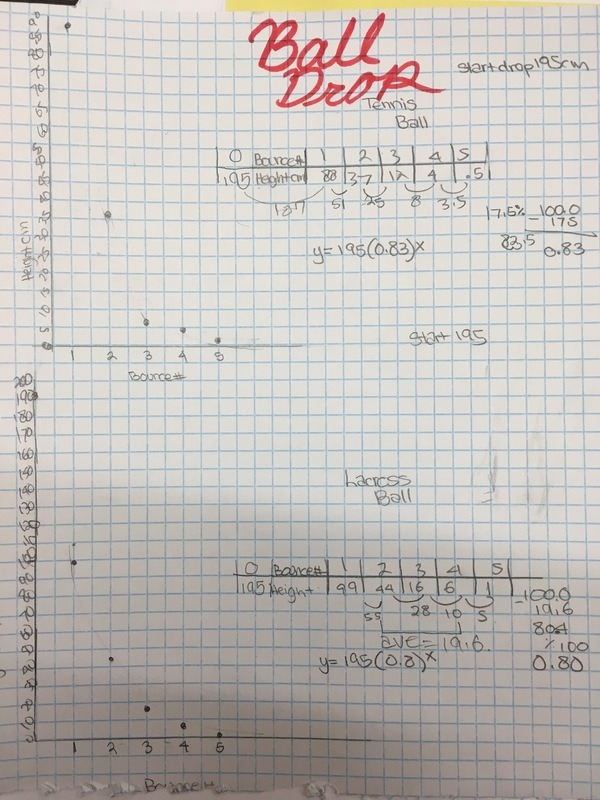









































 RSS Feed
RSS Feed



